

Next: 8 Coupled Channels Methods
Up: Methods of Direct Reaction
Previous: 6 Partial-wave expansions
Subsections
The coupling terms Vij need to be determined for common reaction
mechanisms such as inelastic excitations of nuclei, or particle transfers
from the projectile to/from the target.
7.1 Nuclear Rotational Model
Consider one deformed nucleus with deformation lengths
 ,
(the fractional deformation
,
(the fractional deformation  times some average
radius Rc).
The effect of these deformations can be expressed as a change in the radius
at which we evaluate the optical potentials, the change depending on the
relative orientations of the radius vector to the intrinsic orientation
of the nucleus. When U(R) is the potential shape to be deformed,
the coupling interaction is
times some average
radius Rc).
The effect of these deformations can be expressed as a change in the radius
at which we evaluate the optical potentials, the change depending on the
relative orientations of the radius vector to the intrinsic orientation
of the nucleus. When U(R) is the potential shape to be deformed,
the coupling interaction is
 |
|
|
(42) |
where the `shift function' has the multipole expansion
 |
|
|
(43) |
( is the vector
is the vector  rotated to the
body-centred frame of coordinates defined by
rotated to the
body-centred frame of coordinates defined by  ).
Transforming to the space-fixed frame of reference,
and projecting onto the spherical harmonics,
the multipole expansion becomes
).
Transforming to the space-fixed frame of reference,
and projecting onto the spherical harmonics,
the multipole expansion becomes
The correction  is designed ([8])
to ensure that the volume integral of the nuclear monopole potential
is designed ([8])
to ensure that the volume integral of the nuclear monopole potential
 is the same as that of U(R), and is correct
to second order in the
is the same as that of U(R), and is correct
to second order in the
 .
When the
.
When the
 are small, the above multipole functions
are simply the first derivatives of the U(R) function:
are small, the above multipole functions
are simply the first derivatives of the U(R) function:
 |
|
|
(44) |
with the same shape for all nuclear multipoles  .
The deformations of the Coulomb potential can also be defined by the
.
The deformations of the Coulomb potential can also be defined by the
 , but more accurately by means of the Coulomb reduced
matrix element
, but more accurately by means of the Coulomb reduced
matrix element
 that is directly related to electromagnetic decay
strengths as
that is directly related to electromagnetic decay
strengths as
 .
The reduced matrix element defined by the Wigner-Eckart theorem of the form.
.
The reduced matrix element defined by the Wigner-Eckart theorem of the form.
 |
(45) |
For a rotational model of the nucleus, the matrix element is determined
to first order in
 by
by
 |
|
|
(46) |
for transitions from a state of spin I to one of spin I'
in a rotational band of projection K in a nucleus of charge Z.
The radial form factors for Coulomb inelastic processes are
derived from the multipole expansion of
 , giving for interactions with the
other nucleus (charge Z') of
, giving for interactions with the
other nucleus (charge Z') of
 |
|
|
(47) |
Note that, especially for dipole and quadrupole couplings ( ),
these Coulomb couplings have a long range that is much larger than the
sum of the radii of the interacting nuclei. Any method for numerically
solving the coupled equations with these couplings has to include
some particular treatment of these couplings at large distances
as discussed in ref. [16].
For projectile inelastic excitations, the coupling matrix elements
between different partial waves defined with Eq. (30) are
),
these Coulomb couplings have a long range that is much larger than the
sum of the radii of the interacting nuclei. Any method for numerically
solving the coupled equations with these couplings has to include
some particular treatment of these couplings at large distances
as discussed in ref. [16].
For projectile inelastic excitations, the coupling matrix elements
between different partial waves defined with Eq. (30) are
 |
|
|
(48) |
whereas for target excitations,
having defined the `spatial' couplings as
The rotational model factor
 has been built into the definition of the Coulomb reduced matrix element.
has been built into the definition of the Coulomb reduced matrix element.
7.2 Rearrangement Reactions
If the nuclear state 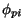 is transformed into state
is transformed into state  by removal of some nucleon(s), then we can define an overlap wave function
by removal of some nucleon(s), then we can define an overlap wave function
 |
|
|
(50) |
The partial wave components of this overlap can be written as the sum of some amplitudes
A times normalised wave functions  . The coefficients A are called
spectroscopic amplitudes (or coefficients of fractional parentage),
and their square moduli |A|2 the spectroscopic factors.
If a coupling order
. The coefficients A are called
spectroscopic amplitudes (or coefficients of fractional parentage),
and their square moduli |A|2 the spectroscopic factors.
If a coupling order
 is used,
the composite nucleus wave function is
is used,
the composite nucleus wave function is
![$\displaystyle \phi_{JM} ( \xi_{pj} , {\bf r}) = \frac{1}{\sqrt{n_{pi}}}\sum_{\e...
...}^{jIJ} ~
\left [\phi_I(\xi_{pj}) \varphi_{\ell sj} ({\bf r}) \right ]_{JM} \ ,$](img176.gif) |
|
|
(51) |
and
 |
(52) |
is the spectroscopic factor. The npi is again the number of nucleons
(or clusters) in the composite system 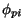 that are identical to
that transferred, and the npi-1/2 factor arises
because of the normalisation of antisymmetrised wave functions for the core
and composite nuclei. (In many common reactions with or near closed shell nuclei,
the npi-1/2 factor cancels some of the n! terms in eqs. (19,21)).
Similar target overlap wave functions can also be defined.
To calculate the coupling term that arises when a particle is transferred,
for example from a target bound state to being bound in the projectile,
we need to evaluate matrix elements
where the initial (primed) state has a composite target
with internal coordinates
that are identical to
that transferred, and the npi-1/2 factor arises
because of the normalisation of antisymmetrised wave functions for the core
and composite nuclei. (In many common reactions with or near closed shell nuclei,
the npi-1/2 factor cancels some of the n! terms in eqs. (19,21)).
Similar target overlap wave functions can also be defined.
To calculate the coupling term that arises when a particle is transferred,
for example from a target bound state to being bound in the projectile,
we need to evaluate matrix elements
where the initial (primed) state has a composite target
with internal coordinates

 and the final (unprimed) state has a composite projectile
with internal coordinates
and the final (unprimed) state has a composite projectile
with internal coordinates

 Let
Let  be the interaction potential,
of which the prior form is
be the interaction potential,
of which the prior form is
 |
|
|
(53) |
and the post form is
 |
|
|
(54) |
where
 is the potential which binds
is the potential which binds

 are the optical potentials, and
are the optical potentials, and
 is the `core-core' potential, here between the p'
and the t nuclei.
The
is the `core-core' potential, here between the p'
and the t nuclei.
The  will be real, but the
will be real, but the  and
Ucc will typically have both real and imaginary components.
The matrix element is now a non-local
integral operator, as it operates on the function
and
Ucc will typically have both real and imaginary components.
The matrix element is now a non-local
integral operator, as it operates on the function
 to produce a function of R.
This section therefore derives the non-local kernel
to produce a function of R.
This section therefore derives the non-local kernel
 so that the matrix element operation on a wave function,
which initially involves a five
dimensional integral over
so that the matrix element operation on a wave function,
which initially involves a five
dimensional integral over  and
and  ,
may be calculated by means
of a one-dimensional integral over R':
,
may be calculated by means
of a one-dimensional integral over R':
 |
|
|
(55) |
Note that when the initial and final single-particle states are real, then
the kernel function is symmetric
 |
|
|
(56) |
When the potential V contains only scalar potentials, the
kernel calculation can be reduced to the problem of finding
the spatial part
 defined so that, given
defined so that, given
the integral operator
 has the kernel function
has the kernel function
 Now the
Now the  and
and  are linear combinations of the channel vectors
are linear combinations of the channel vectors
 and
and  :
:
 and
and
 where,
when
where,
when
 is the projectile bound state,
is the projectile bound state,
 |
|
|
(58) |
with
 ,
,
 ,
and
,
and
 .
When
.
When
 is the target bound state
is the target bound state
 |
|
|
(59) |
with
 ,
,
 ,
and
,
and
 .
The `core-core' vector is always
.
The `core-core' vector is always
 Thus the spherical harmonics
Thus the spherical harmonics
 and
and
 can be given in terms of the spherical harmonics
can be given in terms of the spherical harmonics
 and
and
 by means of the Moshinsky[9]
solid-harmonic expansion (see also refs. [10,11])
by means of the Moshinsky[9]
solid-harmonic expansion (see also refs. [10,11])
from
 , where
, where
We now perform the Legendre expansion
 |
|
|
(62) |
where the Legendre polynomials PT (u)
are functions of u, the cosine of the angle between
 and
and  by using
r = (a2 R2 +b2 R'2 + 2abRR' u)1/2
(with r' analogously) in the numerical quadrature of the integral
by using
r = (a2 R2 +b2 R'2 + 2abRR' u)1/2
(with r' analogously) in the numerical quadrature of the integral
 |
|
|
(63) |
Using the Legendre expansion, the radial kernel function
These formulae can also be used with
 to calculate the kernel
functions
to calculate the kernel
functions
 for the wave function overlap operators
for the wave function overlap operators
 needed in evaluating the non-orthogonality terms of section 8.1.
When the projectile wave functions
needed in evaluating the non-orthogonality terms of section 8.1.
When the projectile wave functions
 are all s-states (
are all s-states ( and
the interaction potential is of zero-range
and
the interaction potential is of zero-range
 then the form factor
then the form factor
 of equation (65) can be simplified to
of equation (65) can be simplified to
This can be made local by defining a new step size
 in the stripping channel
in the stripping channel  .
If the interaction potential is of small range, though not zero,
and the projectile still contains only s-states,
then a first-order correction may be made to the above form factor.
This correction will depend on the rate of oscillation of the source wave
function
fJT (L' J'p),J' ,J't (R' )
within a `finite-range effective radius'
.
If the interaction potential is of small range, though not zero,
and the projectile still contains only s-states,
then a first-order correction may be made to the above form factor.
This correction will depend on the rate of oscillation of the source wave
function
fJT (L' J'p),J' ,J't (R' )
within a `finite-range effective radius'  .
The rate of oscillation is estimated from the local energy
in the entrance and exit channels,
and the result[12]
is to multiply
.
The rate of oscillation is estimated from the local energy
in the entrance and exit channels,
and the result[12]
is to multiply
 in the previous section by a factor
in the previous section by a factor
![$\displaystyle \left [ 1 + \rho^2 {2 \mu _\alpha^{(p)}\over \hbar^2} ~
\left ( U...
...'} ( R) + V_{\ell' sj'} (R)
-U _\alpha (R) + \epsilon _\alpha \right ) \right ]$](img247.gif) |
|
|
(66) |
where the U(R) are the optical potentials, with
 the single-particle binding potential in the target.
The
the single-particle binding potential in the target.
The
 is the reduced mass of the particle in the projectile,
and
is the reduced mass of the particle in the projectile,
and
 its binding energy.
At sub-Coulomb incident energies[13], the details of the nuclear potentials
in equation (66) become invisible, and as the longer-ranged Coulomb
potentials cancel by charge conservation, the form factor
can be simplified to
its binding energy.
At sub-Coulomb incident energies[13], the details of the nuclear potentials
in equation (66) become invisible, and as the longer-ranged Coulomb
potentials cancel by charge conservation, the form factor
can be simplified to
![$\displaystyle u_{\ell' sj'} (R) \ \ D_0
\left [ 1 + \rho^2 {2 \mu _\alpha^{(p)}\over \hbar^2} \epsilon _\alpha \right ]$](img251.gif) |
= |
 |
(67) |
where
![$\displaystyle D = D_0 \left [ 1 + \left ( \rho k _\alpha^{(p)} \right ) ^2 \right ]$](img253.gif) |
|
|
(68) |
is the effective zero-range coupling constant for sub-Coulomb transfers.
The parameters D0 and D can be derived
from the details of the projectile bound state
 .
The zero-range constant D0 may be defined as
.
The zero-range constant D0 may be defined as
 |
|
|
(69) |
The parameter D, on the other hand, reflects the asymptotic
strength of the wave function u0ss (r) as
 ,
as it is the magnitude of this tail which is important in sub-Coulomb reactions:
,
as it is the magnitude of this tail which is important in sub-Coulomb reactions:
 |
|
|
(70) |
It may be also found, using Schrödinger's equation, from the
integral
 |
|
|
(71) |
From this equation we can see that as the range of the potential becomes smaller,
D approaches D0. The `finite-range effective radius'
 of equation (68) is thus some measure of the mean radius
of the potential V0ss(r).
of equation (68) is thus some measure of the mean radius
of the potential V0ss(r).


Next: 8 Coupled Channels Methods
Up: Methods of Direct Reaction
Previous: 6 Partial-wave expansions
Prof Ian Thompson
2006-02-08







![$\displaystyle ~~ \left [ {\sf V}_\lambda^C (R) +
\hat{I'} \langle I'K\lambda 0\vert IK\rangle {\sf V}_\lambda^N (R) \right ]$](img171.gif)
![$\displaystyle \phi_{JM} ( \xi_{pj} , {\bf r}) = \frac{1}{\sqrt{n_{pi}}}\sum_{\e...
...}^{jIJ} ~
\left [\phi_I(\xi_{pj}) \varphi_{\ell sj} ({\bf r}) \right ]_{JM} \ ,$](img176.gif)






![$\displaystyle u_{\ell' sj'} (R) \ \ D_0
\left [ 1 + \rho^2 {2 \mu _\alpha^{(p)}\over \hbar^2} \epsilon _\alpha \right ]$](img251.gif)


